21
Thinking at the Small-Group Unit of Analysis
After I had put most of this book together, I offered a CSCL seminar at Drexel University in the Spring of 2003 based on the manuscript. The responses from students of different backgrounds underscored for me how hard it was for most people to accept the notion of group cognition. I subsequently wrote the introduction and chapters 19 and 20 to scaffold the cognitive change that I was asking of individual learners and of groups of readers.
In preparing for a European CSCL symposium a few months later, I decided to try to present an overview of my research through the lens of the notion of group cognition that emerged in the book. I wanted to stress the empirical basis of this work, so I showed several video clips and transcripts, including the old SimRocket clip and data from my current VMT project. In putting these data sets next to each other, I realized that they showed nicely complementary examples of higher-order scientific and mathematical thinking at the small-group unit of analysis. I presented the same talk at several European research labs and had stimulating discussions that led me to expand and clarify various points. I then included the presentation as the final chapter of this book.
This chapter wraps up the view of group cognition that emerged in the previous chapters. In particular, it discusses the approach of taking the collaborative group rather than the individual group member as the unit of analysis in making visible knowledge-building achievements. This is largely a methodological concern: how can researchers analyze and understand collaborative learning and cooperative working, particularly when it is computer supported?
Consideration of this issue suggests a research agenda for going forward from the preceding studies. Initial observations from work that continues beyond the scope of this book are presented here to point the way for further exploration of the issues raised. The book ends by indicating the kind of empirical investigation needed to explore the world of group cognition that has been opened in the book.
The title of this chapter can be interpreted in various ways, including:
Š Methodological: How should researchers be thinking? Should their thinking be focused on what is happening at the small-group unit of analysis?
Š Analytic: What is the nature of group thinking? Can what takes place at the small-group unit of analysis really be considered thinking?
Š Theoretical: Is this notion of group cognition an innovative idea? Is thinking at the small-group unit of analysis a new conceptualization of distributed cognition?
All three of these senses will be pursued in the chapter as a way of gathering together what has emerged in the book about group cognition. First, I will argue that CSCL researchers should focus less on the individual and more on the group as agent of collaborative knowledge building. Then I will anticipate the kind of research that I think is needed in the future by analyzing an empirical instance of group cognition in a computer-supported collaborative learning context and argue that the group there is engaged in mathematical argumentation and problem solving. Finally, I will conclude by briefly discussing the relation of the concept of group cognition as it has emerged here to recent notions of distributed cognition.
How Should We Understand Collaborative Learning?
Considered in the terms presented in chapter 20, this book has moved from concrete case studies of technology to abstract conceptual reflections. It is now time to use the theoretical framework of group cognition to return to empirical data, and to use the theory for developing a rich understanding of the data. Then, conversely, detailed empirical applications of the theory can serve to clarify and refine the conceptual framework.
In this final chapter, I want to share some initial glimpses into the data we are starting to collect in the Virtual Math Teams (VMT) project as a way of indicating a direction for future research. Up to this point, the book has primarily presented studies of software, analysis and theory from before I moved to Drexel University in September of 2002. Since then, I began the VMT project (introduced at the end of chapter 17) with colleagues at Drexel. This project is concerned with investigating how students can collaborate on mathematical problem solving with the help of technologies like chat rooms. The research takes as its primary unit of analysis the collaborative small group, its group discourse and its group cognition. By investigating collaborative math problem solving, the VMT project targets the analysis of high-level cognitive accomplishments by small groups of students.
It should have already become clear that the focus on the group unit of analysis is not predominantly meant as an ontological commitment to group mind as some kind of physical or metaphysical thing. Rather, it is an alternative way of looking at subtle linguistic interactions that our preconceptions and our languages are not capable of grasping readily as such. In the Heideggerian imagery of chapter 20, group cognition is not a being, but the form of Being of a group, in which a network of semantic references are gathered together to form a coherent meaning.
The focus on the group is largely an attempt to de-focus the individual—recognizing that the thinking individual is really a complexly mediated process, a product of social and cultural forces, despite its visual appearance as associated with a simple physical entity. To exclusively consider the individual and to treat the social interactions that involve the individual as secondary environmental influences (Vera & Simon, 1993) is problematic enough in learning theory generally. But when analyzing technologically mediated collaboration, such a strong focus on individuals necessarily misses and/or under-values interactional and group-level phenomena.
Collaboration itself is an essentially group-level phenomenon, not an individual behavior or attribute. Of course, it is possible to define CSCL as an area of learning research and to adhere to the traditional conceptions and methodologies oriented toward individual learners—merely measuring the effects of computer-based tools and collaborative experiences on the learning outcomes of individual subjects—as was generally done in social psychology, cooperative learning and small-group studies in the past (Johnson & Johnson, 1989; Stahl, 2000). But this book has tried to take CSCL seriously as a new paradigm of theory and research rigorously centered on collaboration. It has tried to affect a shift in thinking and observing. The reflections in the previous chapters of part III, in particular, have been designed to critique the exclusive consideration of individual-level phenomena and to overcome widespread initial resistance to thinking at the small-group unit of analysis.
The recommendation of prioritizing the group unit is primarily a methodological suggestion, rather than an ontological commitment. It hypothesizes that it will be productive to analyze collaborative sense making—the creation of shared meaning—as something that takes place at the group level, as a form of group cognition. Because it is centrally concerned with promoting collaborative knowledge building (see chapter 17), CSCL should try to comprehend meaning making at the small-group unit of analysis and should design software to support group cognition as such.
What is meant here by a methodological recommendation? A basic methodological question for the daily practice of CSCL research is “how can we analyze instances of collaborative learning?” For instance, if we have a video clip or chat log of students collaborating, how do we go about determining what they are doing and what they are learning? If we say that the group members have certain “methods” for accomplishing whatever it is they are doing, then what are our methods as researchers for identifying and analyzing their methods? Ultimately, researchers in CSCL want to understand, evaluate and re-design educational interventions and technological media. If we are using a design-based research approach to do this, then what analytic methods should we incorporate in this approach to understand and critique the learning practices that take place under various conditions?
In particular, if we are investigating learning under conditions of collaboration, we need to have methods to analyze both the individual and the group levels of description. In analyzing group-level behaviors, we can treat the group as the sum of its members and analyze it from the individual perspective, but we can also view the group as an emergent phenomenon with its own set of ideas and behaviors.
Multiple Units of Analysis
The tradition in educational and psychological research has been to focus primarily on the individual person as the unit of analysis. Group behavior is seen as the interplay of actions that are ultimately understood as the behavior of the individual members. In these traditions, the questions that the researcher poses when looking at data might be: What is this or that individual person doing, thinking, intending or learning?
In sociology or anthropology, by contrast, methodology focuses on the social unit or the shared culture as the unit of analysis. Researchers in these traditions might ask: What are the norms, institutions, values, rules or methods that dominate behavior in the society or culture of the studied group?
This book has proposed a middle ground between the extremes of individual and society: the small group as unit of analysis. It suggests that fields like CSCL and CSCW that are concerned with collaborative learning or cooperative work should focus their empirical analysis on what is happening at the small-group level of description. Remember that the small group does not primarily exist as a set of physical objects (the bodies of the members) but as a shared discourse (where discourse may importantly include gaze, physical gesture, body posturing and intonation, or it may be computer mediated in a virtual space). Chapter 20 talked about this as looking at how language speaks through us rather than how we speak using language—viewing the discourse as the site of agency. Key questions here might be: What is taking place interactionally; how are particular utterances contributing to the group discourse; how is shared meaning being constructed?
Conversation analysis based on ethnomethodology adopts a phenomenological stance. Following the method of Husserl—founder of phenomenology and teacher of Heidegger—this approach restricts itself to the given data, without relying upon theoretical constructs and inferences. In analyzing a chat log, we are only justified in interpreting the posted texts, as sequentially given. We cannot speculate on intentions, mental models or internal representations of the participants except insofar as these are expressed or made relevant in the sequential texts themselves. Other scientific approaches might argue from theories of human behavior, even from theories of neuron firings, cognitive loads, sociological forces, etc.
As noted in chapter 18, chat participants are typically also restricted to interpreting the chat on the basis of the temporally appearing sequence of posted texts. That puts the researchers on a non-privileged equal basis with the group members. The interpretation by the researcher relies on a hermeneutic competence shared with the subjects, but takes advantage of a non-engaged, reflective attitude and the opportunity to repeatedly review the log.
Since the meaning of the indexical, elliptical, projective texts in the log spans multiple texts contributed by multiple participants and constituting a small-group interaction, it is appropriate to interpret the log primarily at the small-group unit of analysis. Individual utterances are fragmentary; to ascribe meaning to them one must either see them as part of the larger group interaction or else as expressions of mental representations or mental models (e.g., models of the topic under discussion, of the other participants or of the group process). But mental representations are not visible; they are speculative constructs of the researcher based on psychological or folk theories. It is often tempting to assume some individual intentions or mental states causing the utterances that are observable—because we are so familiar with the use of these theoretical constructs in giving retrospective accounts of human behavior. But these construals go significantly beyond the evidence given in conversational data. Conversation analysis discusses what is happening interactionally within the group discourse; it does not make causal claims about why participants are doing their individual actions that produce the group interaction.
It may often be possible to reduce the analysis of an interaction in a small group to a series of actions of individuals, each responding to what they perceive and interpret. This is particularly plausible when the interaction has not effectively constituted a functional knowledge-building group, but remains at the level of several individuals exchanging their own personal opinions. However, when deep collaborative learning takes place within a group that has been constituted by its participants as the subject or agent of knowledge building, then the group cognition has properties that are different than the sum of the individual cognitions. In general, collaboration is an interactive phenomenon with meaning-making properties at the group unit of analysis. These important properties are easily lost through methods that focus on phenomena at the individual unit of analysis.
To analyze small-group discourse one must be prepared to integrate many considerations. An utterance gathers together previous utterances, it references artifacts and it projects future activity possibilities. So the utterance’s topic implies a much broader, potentially unbounded target for interpretation involving an interplay between (a) individual and (b) community, figure and ground, text and context. (a) On the one hand, specific utterances are primarily contributed by individual speakers (although sentence completions of one person’s utterance by another person are common and all utterances must be understood as responses to the group history and situation). So the small group obviously incorporates its individual members, and one can attempt to reduce one’s interpretation of each utterance to the psychological state of its individual speaker (as secondarily influenced by the other speakers). (b) On the other hand, discourse takes place within the language of a culture, and the content of discourses typically reference social and cultural issues and traditions. How we interact in general is a cultural matter. So the small-group discourse is essentially embedded in a much larger socio-cultural context that must be taken into account when analyzing the small-group unit.
Although the individual, group and community levels are inextricably intertwined, it is useful for purposes of analysis to distinguish them explicitly. Table 21-1 summarizes the five perspectives that have played a major role in this book and that were defined in the beginning of chapter 18.
Table 21-1 goes approximately here

The first three perspectives in the table are those of the agents at the different units of analysis just discussed: the individual, small group and community of practice. The other two perspectives are those of research teams, where the perspectives of analysts who are describing what takes place in their data can be distinguished from the perspectives of educational innovators when they are oriented toward design issues involving curricular or technical interventions. These perspectives are different viewpoints for analyzing empirical interactions; they signify alternative knowledge-constitutive human interests (Habermas, 1965/1971); they allow for making different sorts of analytic or interpretive claims about what is taking place in the data.
Each perspective has its own vocabulary of technical terms corresponding to the interests of that perspective. Chapter 16 proposed that we talk about meaning making taking place at the small-group level, based upon interpretations of the shared meaning that take place at the individual level. To that distinction we can add that the meaning making takes place through methods that enact social practices which have been defined and are shared at the level of communities of practice. Analysis and design take place within the activities of different phases of design-based research cycles (Design-Based Research Collective, 2003). These distinctions and their associated analytic vocabularies allow us to avoid confusions. For instance, if we do not confine talk of meaning making to the group level, then the term “meaning” could get construed in very different ways by different people—as mental content in individual heads, as shared understanding of groups and as widely accepted conventions in communities—causing misunderstanding and arguments based on people making different assumptions (interpretations) about what is being discussed (meanings).
Groups Rock!
Groups are where the meaning-making action is; they are the engines of knowledge building. Accordingly, this book has come to recommend that analysis of collaboration focus on the small group. The small group is seen as an intermediate level of description between that of the individual and the community. Let us see how the prominent theories of collaboration conceptualize the role of small groups.
One can distinguish three general approaches to a theory of collaborative learning in CSCL:
(1) Vygotsky’s (1930/1978; 1934/1986) theory is often read as focusing on the psychology of the individual; internalization of the social by the individual is the key form of mediation.
(2) Lave’s theory (1988; 1991; Lave & Wenger, 1991; 1996), in contrast, is read as a sociological focus on the community, where mediation takes place primarily through changing participation patterns within social practices.
(3) This book has tried to outline a theory at the intermediate level, in which small groups mediate between the individual and larger social formations.
In a sense, the central role of small groups is implicitly acknowledged in studies by both Vygotsky and Lave. Vygotsky’s (1930/1978) analysis of the infant’s grasping/pointing gesture discussed in chapter 16 treats the mother/infant dyad as a small group in which the interpretive perspectives of the two individuals are dissolved in a process of shared meaning making. Similarly, the apprenticeship studies reported by Lave and Wenger (1991) all involve small groups, typically consisting of one mentor and several apprentices or even just a small group of apprentices who learn together. In these examples, the small group mediates between the individual learning and social institutions of professional language and economic production.
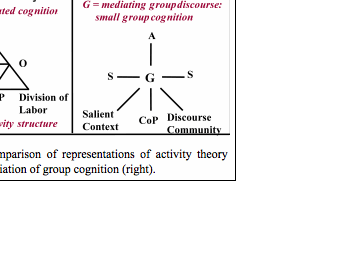
The same point can be made about activity theory studies (e.g., those in Engeström, Miettinen, & Punamäki, 1999; Nardi, 1996). In order to discuss communities of practice, such studies actually analyze behavior in small groups—for instance Engeström’s discussions of “knotworking” as fluidly changing group formations (Engeström, Engeström, & Vähäaho, 1999). However, the theory itself does not acknowledge the crucial role of small groups. For instance, Engeström (1999) stresses the importance of conducting analyses at both the individual and community unit of analysis and of relating these to each other, but he does not emphasize the intermediating role of the group unit of analysis. Furthermore, his popular representation of activity theory’s extended triangle of mediations fails to represent the group, despite the central role of small groups within the concrete studies. Figure 21-1 contrasts the activity theory triangle with a simple representation of the mediation of group cognition.
Figure 21-1 goes approximately here
On the left side of figure 21-1, we see how the idea of a relatively simple perceptual or communication channel connecting a subject and an object (Shannon & Weaver, 1949) was supplemented by Vygotsky (1930/1978) to include the mediation by a physical or symbolic artifact, and then extended by Engeström (1999) to include a layer of socio-cultural mediations. On the right, a representation of a dialogical relation of two (or more) subjects connected by discourse is supplemented to explicitly indicate the mediation by their group discourse, and then extended to include artifacts, a community of practice, a discourse community and other contextual features made salient in their discourse. The point of the contrast is that the activity theory representation fails to acknowledge the role of the small group, group discourse, or group cognition as a central agent of mediation. This book’s contribution is to make the role of small groups explicit and prominent, and to begin to develop an associated concept of discourse as group cognition.
If we want to advance the third alternative for a theory of collaborative learning, here are some research hypotheses for future empirical investigation and for a theory of small-group cognition grounded in such analysis:
Š The small group is the primary unit that mediates between individual learning and community learning.
Š Community participation takes place primarily within small-group activities.
Š Individual learning is acquired largely through participation in these small-group activities.
Š Individual identities are formed and acknowledged through small-group activities.
Š Community practices are enacted and reproduced through small-group activities.
These hypotheses motivate my latest research.
Varieties of Collaboration
Having proposed the centrality of small groups and articulated the concept of group cognition, the next step is to use this to guide empirical analysis of actual instances of collaboration. The Virtual Math Teams project was designed to begin this effort. It started by gathering examples of collaboration under different conditions. Although the VMT project has only completed the first of its five years, the VMT team is already observing instances of group cognition in its data. We have not yet undertaken thorough analysis of this data guided by the theory in this book, but we can begin to see the richness of such data from this perspective.
Our first experiment was to go into a middle school classroom in an urban public school and videotape a collaborative math problem-solving lesson. The VMT project is concerned with collaboration that is neither face-to-face nor tied to a school context; however, we wanted to start with this kind of traditional experience as a baseline for understanding—by analogy and by contrast—what takes place in the virtual setting.
We videotaped four girls sitting around a table in the classroom and working on a task. The collaboration that took place was very different from that of the five boys with the SimRocket simulation discussed in chapters 12 and 13. The girls sat facing each other, but each worked on their own piece of paper. They each pursued the assignment themselves, despite the fact that the group’s explicit assignment had been to produce one paper to hand in and that they were supposed to take different roles in producing that paper. As they worked, they softly spoke out loud—but as if thinking to themselves—about what they were doing. They counted aloud, complained in frustration that they were confused (“lost”), or expressed pain (“headache”). They took turns looking up to see how the others were doing and to review each other’s progress on their papers. This running commentary on their problem solving provided a continuous social intercourse. They periodically judged themselves and each other as smart or lost, articulating social and intellectual identities. When one person was particularly lost, someone would point at that person’s paper and help them through the computation step by step. It was clearly important to all the participants that everyone maintain pace and complete each major phase of the task before the others could continue.
In this case study, collaboration took place through highly synchronized parallel work. We might term this “parallel,” “indirect” or “multivocal” collaboration (Cobb, 1995, pp. 42f). This was a different form of collaboration than in the SimRocket case. In the latter case, the boys worked as a group and solved the task jointly within a single discourse. The intense collaborative moment that they engaged in was concentrated on making sure that each of the participants had the same understanding about which rockets were being discussed and that everyone agreed that they were different in the relevant sense. In the case of the girls, they looked like they were working individually, although closer inspection showed that they also put considerable effort into ensuring that they all had the same results and a similar understanding. The relationship of the group work to the individuals was different. Where the boys’ utterances alternated so that the group discourse was a sequence of sequential statements that built into one line of argument, the girls’ talk proceeded more in parallel, seemingly independently, although they did respond to each other’s comments and re-used each others’ terms and phrases. In both cases, the end result was that the task was accomplished through a blending of contributions from all the participants—what chapter 7 called intertwining of perspectives—and everyone shared the same basic understanding of what had been accomplished.
In the VMT project, we have begun to characterize the difference between the way the group of boys collaborated and how the girls did as a difference of exploratory versus expository knowledge building. We notice this alternative as a quite general pattern, with some groups switching between the two methods during a single session. In exploratory collaboration, the group investigates proposed lines of inquiry together, as the group of boys did. In expository processes, one member at a time narrates their finding or solution, explaining and justifying it enough to garner agreement, much as the girls did. The difference in these two group methods is apparent in both quantitative (coding statistics) and qualitative (conversation analytic interpretations) comparisons of video transcripts as well as of chat logs. It was also anticipated in Bereiter’s quote in section 1.2 of chapter 15.
In other face-to-face experiments, ten groups of three or four students working together on a relatively open-ended geometry problem were videotaped. Although the styles of interaction of these groups were extremely diverse, they all included patterns of proposals being offered for consideration and then being discussed, checked, critiqued and either accepted, rejected or modified. This is a process that was called negotiation in part I, especially in chapter 8. Negotiation methods are ways in which contributions from individuals can be accepted into a group’s shared knowledge. This gives group members opportunities to react to the contribution and to influence what is adopted. It thereby involves the group members in the development of ideas and keeps the individuals synchronized with the group and with each other—to the extent required and in the ways needed for the practical purposes of the group discourse and group interaction. The negotiation process can be conducted through a broad range of methods, with members adopting various implicit or explicit roles.
The way in which group discourse grows through negotiation provides for its potential strength. Through proposals by many members, the group discourse incorporates and benefits from insights from multiple personal perspectives (backgrounds, interests, skills, emphases). By means of checking one person’s proposal with others from their different perspectives, proposals are subjected to critical review, and weaknesses can be uncovered and corrected. In such ways, the group cognitive process gathers together strengths of the individual minds and can overcome some of their weaknesses.
In the data we have started to collect, we can begin to see how small groups actually negotiate shared knowledge. Analysis of this data should inform our understanding of how to design software to support knowledge negotiation and small-group collaboration generally in order to avoid the problems met by the software prototypes discussed in part I of this book. Of course, we particularly need to study methods groups use to negotiate when they are collaborating online.
Chatting about Math
The video data discussed so far recorded face-to-face interactions. However, the Virtual Math Teams project is most interested in computer-mediated online collaboration. We have just begun on a small scale to invite students to a chat room to work on math problems in virtual teams of 2 to 5 students. An excerpt from near the beginning of the chat log from one of our first sessions is shown in figure 21-2.
Figure 21-2 goes approximately here
The math problem for this chat is:
If two equilateral triangles have edge-lengths of 9 cubits and 12 cubits, what’s the edge-length of the equilateral triangle whose area is equal to the sum of the areas of the other two?
From an educational innovator’s or a mathematician’s perspective, the most elegant solution to the problem is to consider the proportions of the triangles. Because the three triangles are all the same shape 2-dimensional figures, their areas will be proportional to each other in the ratio of the squares of any given linear dimension. The two smaller triangles have corresponding sides in the ratio of 9:12, which is 3:4. It is well-known (for instance from the 3/4/5 right triangle) that the squares of 3 and 4 add up to the square of 5. So the three triangles should have sides in the ratio of 3:4:5 or 9:12:15. The answer of 15 might well pop into a mathematician’s head when she sees the problem, based on this consideration of proportions.
Even if one did not have this insight into the problem, the most effective algebraic approach to solve the problem is to use an argument about proportions to avoid computing the numeric length of the altitude. Since the triangles are all the same shape, the ratio of the altitude to the base will be the same in each triangle, some constant k. So h = kb and the formula for the area of the triangle will be:
area = ½ bh
= ½ b(kb)
= (½ k)b2
 Then, given that the sides of the squares are
9, 12, x, and the area of the third equals the sum of the other two:
Then, given that the sides of the squares are
9, 12, x, and the area of the third equals the sum of the other two:
(½ k)x2 = (½ k)92 + (½ k)122
x2 = 92 +122
x = 15
The group of students whose work is excerpted in the chat log segment did not adopt this perspective. Their chat is interesting both for the skillful methods they employed in constructing a problem-solving strategy and for the weaknesses we can identify in their ability to take full advantage of all their collective resources. By seeing how a real group of students actually interact in a specific social, technical and mathematical context, we can understand the collaborative knowledge building activity that we are trying to support and can make use of breakdown phenomena to suggest design improvements to the group formation, math task formulation and software environment.
A first impression of the chat log is that it looks similar to a transcript of students talking. As in the face-to-face SimRocket dialog analyzed in chapters 12 and 13, one can see that the individual contributions to the online chat log are indexical, elliptical and projective. They index features of the problem with which they are situated: “that’s two separate triangles” and they point back to previous postings: “o” or “I know how.” They are elliptical, relying on other postings to round out their meaning: “I see” or “proportions?” They project responses or future activities: “i think we have to figure out the height by ourselves” or “how?” Even at the utterance level, meaning is constructed across multiple postings by multiple contributors. As will be seen by an analysis of the larger problem-solving process, thinking through the mathematics is accomplished by the group as a whole—it is an interactive accomplishment achieved in the group discourse. It is an instance of group cognition. This log is an example of precisely the kind of data that needs to be analyzed in order to see how group cognition works in detail.
Perhaps the first thing one may notice here is a pattern of proposals, discussions and acceptances similar to what takes place in face-to-face discourse. Proposals about steps in solving the math problem are made by Avr in lines 1, 3, 8, 17 and by Pin in lines 20, 27. These proposals are each affirmed by someone else in lines 2, 6, 10, 19, 22, 28, respectively.
Another thing to note is that the turn-taking conventions of face-to-face discourse (Sacks et al., 1974) do not exist in chat in an unmediated way. Chat is a quasi-synchronous medium in which everyone can be typing at the same time, and the messages of other people appear sequentially after the posting has been completely typed and sent (Garcia & Jacobs, 1998). In larger groups, this can result in considerable confusion. Even in the small group of three members in this example, one must be careful to check timestamps when interpreting the threading of postings because they do not always follow each other immediately in a simple response sequence the way they do in conversation, which is constrained by the turn-taking patterns of face-to-face discourse. Thus, line 21 responds to line 19, while line 22 responds to line 20. The timestamps show that lines 20 and 21 effectively overlapped each other chronologically: Avr was typing line 21 before she saw Pin’s line 20. Similarly, lines 24 and the following were responses to line 20, not line 23.
So, online chat is similar to face-to-face conversation in some ways and different in others. The characteristics of the technological medium have specific effects on the mediation of the discourse. It is plausible to expect that groups need to meet most of the same fundamental interactional requirements whether computer-mediated or not. For instance, they need to be able to determine what utterance is a response to what other utterance. The methods for accomplishing this in different media may differ in interesting and consequential ways. Applying a conversation analysis approach to an online environment necessitates determining what are the similarities and differences between chat and face-to-face conversation. In addition, conducting math problem solving in a VMT chat room is not exactly the same activity as engaging in informal social conversation. Students have to officially register for the event and show up at the right time and place. Math topics automatically bring in elements of the school context, even if VMT takes place outside of the institutions of formal schooling. The problems are given to the students in the context of an institutional authority and the students are acting as students, watched over by adults. So there are social as well as technological constraints that mediate the interaction and transform conversational methods.
Many students are accustomed to using chat technology for socializing freely with their peers, and they have to adopt a rather different behavior when participating in a VMT chat room. Their methods of interacting reflect this. According to Garcia and Jacobs (1999), normal conversation defines a baseline system of institutionally unconstrained discourse, which has to be adapted to in discourse contexts that have particular definitions, conventions, constraints, goals or structures. Of course, the fact that chat is a text-based medium, in which brief texts are posted in a temporal sequence, makes it quite different from interactive, embodied talk-in-interaction. The VMT context adds even more differences.
In addition, mathematical discourse has a number of specific features. Some of these are visible in the chat log excerpt displayed above. Methods of making various sorts of mathematical proposals are of central concern. Methods of critiquing and otherwise responding to these proposals require a considerable grasp of math content and conventions. As in any human argumentation, math discussions involve complex techniques of persuasion, one-upmanship and verbal sparring as well as the officially sanctioned math reasoning skills taught in math textbooks. Furthermore, the VMT chats provide a social occasion that is important to the participants; the sociality and identity-building aspects of the interaction are necessary if the students are going to return for future chats. As we shall see below, the social and the mathematical characteristics of interaction may be inextricably fused. A given brief posting may be playing multiple sophisticated roles in the chat; its interpretation by a researcher may be a complex, tricky, multi-layered, open-ended and ultimately ambiguous undertaking.
A Failed Proposal
For instance, try to interpret line 23 where Sup says simply “proportions?” What is this line a response to? Why does it occur at precisely this point in the discourse? What work is it doing—or failing to do—in the discourse? Is it posing a question, or is it making a proposal? If it is a question, what is it asking? If it is a proposal, how is it suggesting using proportions? One approach for a researcher faced with such an ambiguous posting is to see how it is taken up by the group members. In this case, this approach is frustrating for the researcher, because the posting does not seem to be taken up by Sup’s peers at all. First, Avr’s next line overlaps Sup’s with the identical timestamp. It is not completely clear what Avr’s posting refers to, but it is likely a response to Pin’s “draw the altitude”—Avr is apparently having trouble drawing the altitude of a triangle on paper near her computer. The following remarks by Pin continue his discussion thread from line 20. (If we, as researchers, draw an equilateral triangle on our own piece of paper and then draw the altitude of the triangle, we can see that the altitude divides it into two 30/60/90 triangles. The chat software did not include a shared whiteboard for the students to display such a drawing, so it took them quite a while to share this insight later in the chat.) Sup’s contribution on line 23 is lost as a result of its overlap by Avr.
The loss of Sup’s contribution from the discussion is consequential from the analyst’s point of view, considering the mathematics of the problem. As discussed above, a focus on relations of proportionality might have been useful in solving the problem.
The group discourse ignores Sup’s suggestion of using proportions and heads down a different road, one that does not ever reach an answer. One can argue from an educational analyst or software designer perspective that this demonstrates a negative possibility of collaboration, sometimes called “group think,” where the group discourse goes off in a direction that prevents an individual from going in a better direction. In fact, Sup effectively dropped out of the chat for a long time. More than an hour later, after he returned, he symbolically computed the value of the ratio of the altitude to the side and proposed again, “i think it is gunna be in proportions.” Unfortunately, his proposals continued to have little effect on the group problem solving.
Certainly, collaboration is not always perfect or even helpful. It remains an empirical hypothesis that collaboration is even appropriate to mathematical problem solving. Some people have the feeling that math is precisely the kind of thing that is best done in isolated concentration, far from the kind of peer pressure that is felt in a chat environment, where one constantly has to keep up with the flow of other people’s contributions and of the group’s movement. On the other hand, math educators argue that math discourse is important for building deep understanding, as opposed to rote application of formulae and computational algorithms. The resolution of this debate requires detailed analysis of mathematical discourses. We need to determine what methods people actually use—both individually and collaboratively—to “do” mathematics, particularly outside the context of textbook drill and practice exercises (Hall & Stevens, 1995; Lave, 1988).
In conducting our experiments, the VMT project is very aware that a simple chat interface is woefully inadequate for supporting effective collaborative math problem solving and discussion. However, the nature of the inadequacy is not clear and the technical solutions for overcoming it are not obvious. Exploratory inquiry is required. One of the most effective investigatory techniques is to zero in on breakdowns and other problems that arise in practice. That is why phenomena like line 23 should be given careful consideration.
It is important from a design perspective to know why line 23 was ignored, particularly given that it might have led to an effective strategy for the group. We would like to know how promising, but failed, proposals can be supported in the group process so that they can be more effective in the collaborative problem solving.

Sup’s posting has the appearance of a question. Superficially, it has the same form as Avr’s question in line 21: a single word followed by a question mark (see figure 21-3). In line 17, Avr made the proposal, “i think we have to figure out the height by ourselves,” to which Pin responded in line 19 by saying, “i know how.” Avr then asks in line 21, “how?” While she is asking, Pin continues his response in line 20, “draw the altitude,” thereby, in effect, answering Avr’s question before even seeing it. When Avr sees Pin’s answer, she says in line 22, “right”—aligning with him. This is a nice example of close coordination between Pin and Avr, which contrasts with Sup.
Figure 21-3 goes approximately here.
Sup’s line 23: “proportions?” can be interpreted as a question in parallel to Avr’s question in line 21. That is, he could be asking Pin if he plans to use some kind of calculation of proportionality to figure out the height of the triangles. However, line 23 comes too late to simply pose this question because Pin has already announced that his plan is to draw the altitude. Sup could be asking if Pin plans to use proportional reasoning in conjunction with the altitudes of the triangles once they are drawn, but this goes beyond asking a simple question like Avr’s, and in effect it would be making a proposal for a further problem-solving strategy. So, perhaps Sup’s posting should be evaluated as a proposal.
One way of pursuing this interpretation from a conversation analysis perspective is to look at the conversational method used for making the proposal in line 23 and to ask why it was less effective than the other proposals in the chat. Two features of Sup’s method have already been mentioned: (a) its lack of clarity and (b) its bad timing. Several other problems are also apparent.
(a) All the other proposals (1, 3, 8, 17, 20, 27) were stated in relatively complete sentences. Additionally, some of the proposals were introduced with a phrase to indicate that they were the speaker’s proposal (1: “I think we should … ,” 17: “I think we have to … ,” 20: “i know how …” and 27: “i think i got it …”). The exceptions to these were simply continuations of previous proposals: line 3 provided the formula proposed in line 1, and line 8 proposed to “then” use that formula. Line 23, by contrast, provided a single word with a question mark. There was no syntactic context within the line for interpreting that word and there was no reference to semantic context outside of the line. Line 23 did not respond in any clear way to a previous line and did not provide any alternative reference to a context in the original problem statement or elsewhere.
(b) The timing of line 23 was particularly unfortunate. We already noted that it overlapped a line from Avr. Because Avr had been setting the pace for group problem solving during this part of the chat, the fact that she was following a different line of inquiry at the time of line 23 spelled death for an alternative proposal. Pin either seemed to be continuing his own thread without acknowledging anyone else at this point, or else he was responding too late to previous postings. So a part of the problem for Sup was that there was little sense of a coherent group process—and what sense there was did not include him. If he was acting as part of the group process, for instance posing a question in reaction to Pin and in parallel to Avr, he was not doing a good job of it, and so his contribution was ignored in the group process. It is true that a possible advantage of text-based interaction like chat over face-to-face interaction is that there may be a broader time window for responding to previous contributions. In face-to-face conversation, turn-taking rules may define appropriate response times that expire in a fraction of a second as the conversation moves on. In computer-based chat, the turn-taking sequence is more open. However, even here, if a participant is responding to a posting that is several lines away, it is important to make explicit somehow to what they are responding. Sup’s posting does not do that; it relies purely upon sequential timing to establish its context, and that fails in this case.
(c) Sup’s posting 23 came right after Pin’s proposal 20 to “draw the altitude.” Avr had responded to this with posting 22, “right,” but Pin seems to have ignored it. Pin’s proposal had opened up work to be done and both Avr and Pin responded after line 23 with contributions to this work. So Sup’s proposal came in the middle of an on-going line of work without relating to it. In conversational terms, he made a proposal when it was not time to make a proposal. It is like trying to take a conversational turn when there is not a pause that creates a turn-taking opportunity. Now, it is possible—especially in chat—to introduce a new proposal at any time. However, to do so effectively, one must make a special effort to bring the on-going work to a temporary halt and to present one’s new proposal as an alternative. Simply saying “proportions?” will not do it.
(d) To get a response to a proposal, one must elicit at least an affirmation or recognition. Line 23 does not really solicit a response. For instance, Avr’s question at line 21 “how?” called for an answer. It was given by Pin in line 20, which actually appeared in the chat window just prior to the question and with the same time stamp. But Sup’s posting does not call for a specific kind of answer. Even Sup’s own previous proposal in line 10 ended with “right?” which at least required agreement or disagreement. Line 10 elicited a clear response from Avr at line 11, “no,” followed by an exchange explaining why Sup’s proposal was not right.
(e) Other proposals in the excerpt are successful in contributing to the collaborative knowledge building or group problem solving in that they open up a realm of work to be done. One can look at Avr’s successive proposals on lines 1, 3, 8 and 17 as laying out a work strategy. This elicits a response from Sup trying to find values to substitute into the formula and from Pin trying to draw a graphical construction that will provide the values for the formula. But Sup’s proposal in line 23 neither calls for a response nor opens up a line of work. There is no request for a reaction from the rest of the group and the proposal is simply ignored. After no one responded to Sup, he could have continued by doing some work on the proposal himself. He could have come back and made the proposal more explicit, reformulated it more strongly, taken a first step in working on it, or posed a specific question related to it. But he did not—at least not until much later—and the matter was lost.
(f) Another serious hurdle for Sup was his status in the group at this time. In lines 10 through 16, Sup made a contribution that was taken as an indication that he did not have a strong grasp of the math problem. He offered the lengths of the two given triangles as the base and height of a single triangle (line 10). Avr immediately and flatly stated that he was wrong (line 11) and then proceeded to explain why he was wrong (line 13). When he agreed (line 15), Avr summarily dismissed him (line 16) and went on to make a new proposal that implied his approach was all wrong (lines 17 and 18). Then Pin, who had stayed out of the interchange, re-entered, claiming to know how to implement Avr’s alternative proposal (lines 19 and 20) and Avr confirmed that (line 22). Sup’s legitimacy as a source of useful proposals had been totally destroyed at precisely the point just before he made his ineffective proposal. Less than two minutes later, Sup tried again to make a contribution, but realized that what he said was wrong. His faulty contributions confirmed repeatedly that he was a drag on the group effort. He then made several more unhelpful comments before dropping out of the discourse for most of the remaining chat.
Does this mean that Sup was an inferior mathematician or a poor collaborator? Collaboration is a group process, not an individual attribute. One could say that Sup’s low status as mathematician and contributor to the group was produced by the group. Avr is an aggressive leader of the group process, as was evident throughout the hour-long chat log. She is skilled at techniques and conventions of chat as an interactional format. Pin exercised various behaviors to maintain his collaboration with Avr. Sup was considerably less successful at this. It may be possible that Sup has strong mathematical intuitions, but is not skilled at formulating them or at making proposals based on them. Perhaps different forms of group facilitation, group formation or group support would allow him to participate more effectively in collaborative math problem solving and to engage more productively in the production of group cognition. A group process that was more supportive for Sup might allow him to work better as a collaborative mathematician. Vygotsky (1930/1978) argued that a student’s ability to perform within a group was at a higher developmental level than the same student’s ability to perform alone—as measured by the zone of proximal development that separates these distinct capabilities. But the higher ability assumes a properly supportive group process. What takes place (and its analysis) on the individual level is derivative of what takes place (and its analysis) at the group unit.
Sup’s misguided attempt to use the lengths of the two triangle sides as the values of the base and height of a single triangle is not as surprising as it might seem. It is an instance of a strategy that is commonly used by students (Verschaffel, Greer, & Corte, 2000). Many students have been trained in school to solve problems by simply plugging given numbers into an available formula. Often, a school math session will consist of practicing computing a given formula with many sets of values. Avr had presented a formula that called for values of base and height (line 3), so Sup took the two numbers given in the problem statement and proposed using them in the formula just because they were at-hand, perhaps without thinking deeply about their role in the mathematics of the problem (Hall, 2000).
It is also possible that Sup was trying to work with the two numbers as part of his intuition about proportionality of the triangles, not just because they were the only numbers given in the problem statement. As discussed above, the mathematically elegant approach to the problem builds on the proportional relation 9:12. Sup may have felt inclined to build on this relation but been unable to work it out. Perhaps if Avr and Pin had combined their skills at making proposals with Sup’s intuitions, then the group cognition would have solved the problem quickly.
Pin thought through the problem carefully along the shared group strategy approach and drew in the missing altitude that was needed for Avr’s formula of the area of a triangle. He then correctly identified the result as forming a standard 30/60/90 triangle. His next step (line 29) was to ask “so whats the formula.” At this point, he too fell into the school pattern of expecting to apply a standard formula to solve a problem.
These are methods of math problem solving that are reinforced in many math classes: identifying a handy formula and plugging in available numbers. The problems used in the VMT project are typically not problems that can be solved with these rote methods. They generally require reflection upon the structure of the situation and some discussion of how to approach the problem. Note how the problem in this chat could be elegantly solved with the considerations of proportionality described earlier in the chapter.
The method used in arithmetic that must be overcome when students learn algebra is numeric calculation. It was this method that ultimately sidetracked Pin and Avr later in the chat. Rather than keeping their formulas in symbolic, algebraic format, they both insisted on substituting numeric values for square roots and multiplying everything out on their calculators. Particularly because they were working at a distance and not sharing one calculator, they wasted a substantial part of the chat hour arguing over insignificant decimal place values. This became a major point of contention and a power struggle between them. As far as the problem-solving process goes, numeric values made it much harder to solve the problem. As we saw above, maintaining everything in symbolic format allows one to cancel out all of the square roots and multiplicative constants. By engaging in numeric computations, one loses track of the overall strategy and gets bogged down in unnecessary and error-prone detail. As a consequence, the group in this chat never solved the problem.
Chat as Group Cognition
A conversation analysis can highlight the various methods that students engage in, both mathematical and interactional. For instance, it can track the level of abstraction of their discourse. Abstraction is an essential dimension of mathematics, and learning algebra requires significant shifts in cognitive abilities to deal with abstraction (Sfard & Linchevski, 1994). This applies to group cognition as well as individual cognition in beginning algebra. We can make this visible by looking at the language used in postings about math (Sfard, 2002). In the excerpt we are considering, the students move between discussing “a triangle” (line 1) or “proportions” (line 23) in general, specific formulae for specific kinds of triangles (line 29), concrete values (line 10) and numeric computations (later).
Consider line 1: “Okay, I think we should start with the formula for the area of a triangle.” With “Okay,” Avr takes charge of the group discourse and announces that she is making a proposal of a strategy for the group to follow. She says, “I think,” indicating that this is a proposal from her individual perspective, but one that she is sharing with the group. She continues, “we should start,” constituting the group of chat participants as a plural subject, as a group agent that should undertake some action. “With the formula” defines the action as a mathematical task and the strategy to be one that uses a particular standard formula. “The area of a triangle” is an abstraction from the three particular triangles of known and unknown size and shape; it references the completely general triangle figure. “A triangle” is quite abstract, but some things are known about triangles in general, including a formula for their area, which Avr gives in her next posting.
After starting with the abstract concept of “a triangle” and proposing a method for working with it, namely to use the formula for area, Avr proposes in line 8 a step toward making it more concrete by applying the formula to the three specific triangles in the problem to “then find the area of each triangle.” As we have seen, Sup thereupon takes the next step in concretizing by providing concrete candidate values for the abstract variables of the formula, b and h or base and height. The rest of the excerpt remains at the intermediate level of abstraction, making proposals about the particular kinds of triangles in the problem, but not yet supplying numeric values. The proposals reference “two separate triangles,” “the height,” “the altitude,” “a 30/60/90 triangle” and “the formula” (for a 30/60/90 triangle). The exception to this is line 23, where Sup makes the very abstract proposal, “proportions?” Unfortunately, he does not state more concretely which proportions he is referring to. This is another way that Sup displays his inability to join the group: at line 10 he made a proposal that was too concrete and now at line 23 he makes one too abstract.
As we have seen from this preliminary look at an initial chat log, members’ postings constitute the group discourse as such and orient to it as salient. For instance, individuals make proposals to be shared by the group. These proposals are often explicitly presented as the individual’s personal opinion (e.g., lines 1, 4, 17, 19, 26), but acceptance by another group member makes it part of the shared flow of considerations and sets it up for being built upon by anyone in the group. Subsequent postings reference them, identifying them as integral to the group discourse. By prefacing a proposal with something like “I think we should …” (line 1), the proposer indicates that she is a group member and also calls on the rest of the group to respond and confirm (or deny or critique) the proposal. As Lerner (1993) shows, stating the first part of an adjacency pair (like question and answer) that elicits a response from someone else can be directed to a whole group. It thereby constitutes the group, as such, as a participant in the conversation and designates the group as the projected respondent. This means that any of the other group members can take the next turn and respond to the proposal on behalf of the group. This is a pattern that we see repeatedly in the chat excerpt, except for Sup’s failed proposal, which did not succeed in eliciting a response.
Some methods of contributing proposals are effective, others are not. We have seen several problems with Sup’s line 23: (a) its lack of semantic clarity (what is he proposing about proportions?) and its weak syntactic structure (is it even a proposal?); (b) its unfortunate timing in being followed immediately by line 24; (c) its inability to interrupt the on-going work; (d) its failure to elicit a response; (e) the absence of any proposed work to be done; (f) Sup’s history of distracting from the flow of the group problem solving rather than contributing to it; (g) Sup’s lack of alignment with the group in terms of level of abstraction. These may have all contributed to the failure of the posting. Line 23 was simply not properly formulated or presented as a proposal that calls for uptake by the group. At best, it is a suggestion, vaguely offered to the other group members for their consideration. Given the rapid flow of proposals and responses in chat, it is too weak to have an effect on the problem solving and is ignored.
Due to the quasi-synchronous nature of the chat medium, there is a competition to time and to structure postings and social relationships so as to increase the likelihood of the posting being taken up into the group discourse (Garcia & Jacobs, 1998). Line 23 fared poorly in this competition. In considering this empirical case in its concrete context, we have noted some of the considerations involved. Methods for doing math collaboratively integrate skills of text chatting (typing, abbreviating, posting quickly, referencing other postings, etc.), socializing (establishing roles, legitimacy and social relations), formulating proposals mathematically (proper level of abstraction, use of symbols, strategies, explanations) and interacting (making effective proposals, leading the group discussion, eliciting desired responses). We have seen problems along all these dimensions with line 23, compared to line 1, for instance.
Many common sorts of methods of doing math appeared in this chat: selecting levels of abstraction, using formulas, substituting numeric values. The mathematics of the chat-mediated group discourse bears strong resemblance to cognitive processes attributed in the math education literature to individual students working on their own. Students are likely to grasp at available formulae or pursue numeric computations with whatever numbers are at hand. Some of the math methods we observed are simultaneously methods of interacting socially and constituting group identity (e.g., who can play what role, whose proposals will be taken seriously). In particular, leadership in the group interaction seems to be both defined by and controlled by the making of effective math proposals. Avr’s self-identity as a leader in the group is maintained by her attempts to control the proposal making and is reflected by her success in making effective proposals that set the group agenda.
Design Support for Group Cognition
In the chat excerpt involving Avr, Pin and Sup, we have seen several examples of a three step pattern:
1. A proposal for the group to work on is made by an individual: “I think we should ….”
2. An acceptance is made on behalf of the group: “Ok,” “right”
3. There is an elaboration of the proposal by members of the group. The proposed work is begun, often with a secondary proposal for the first sub-step.
This suggests that collaborative problem solving of mathematics may often involve a particular form of what conversation analysis refers to as adjacency pairs (like question/answer pairs). We can define a math proposal adjacency pair with this structure:
1. An individual makes a proposal to the group for the group’s work.
2. Another member of the group accepts or rejects the proposal on behalf of the group.
Many adjacency pairs allow for recursive insertion of other pairs between the two parts of the original pair, delaying completion of the pair. With math proposal adjacency pairs, the subsidiary pairs seem to come after the completion of the original pair, in the form of secondary proposals, questions or explanations that start to do the work that was proposed in the original pair.
The centrality of math proposal adjacency pairs in collaborative math problem solving argues for the importance of analyzing thinking at the small-group unit. If one focuses just on individual postings as speech acts, then one is tempted to reduce them to expressions of individual cognitions. As Duranti (1998) critically remarks, “In general, speech act theory takes individual speech acts produced by individuals as its unit of analysis” (p. 253). In contrast, math proposals elicit responses and further work by the group. “Adjacency pairs are thus important mechanisms for establishing intersubjectivity, that is mutual understanding and coordination around a common activity” (p. 255; emphasis in original). Initiated by individuals, math proposals constitute the group as the agent to accept the proposal as shared, and to do the problem-solving work as a group activity. The proposal/acceptance pair is itself the linguistic accomplishment of at least two members of the group and is clearly situated in the activity of the group as a strategy for continuing that group’s activity. Successful adjacency pairs are group accomplishments, not individual acts.
At this time, the notion of math proposal adjacency pairs is just a preliminary proposal based on a brief chat log excerpt. It calls for extensive conversation analysis of a corpus of logs of collaborative online math problem solving to establish whether this is a fruitful way of interpreting the data. If it turns out to be a useful approach, then it will be important to determine what interactional methods of producing such proposals are effective (or not) in fostering successful knowledge building and group cognition. An understanding of these methods can guide the design of activity structures for collaborative math.
For instance, if the failure of Sup’s proposal about proportions is considered deleterious to the collaborative knowledge building around the triangles problem, then what are the implications of this for the design of educational computer-based environments? One approach would be to help students like Sup formulate stronger proposals. Presumably, giving him positive opportunities to interact with other students, like Avr and Pin, who are more skilled in chat proposal making would provide Sup with models and experiences that he can learn from—assuming that he perseveres.
Another approach to the problem would be to build functionality into the software and structures into the activity that scaffold the ability of weak proposals to survive. As students like Sup experience success with their proposals, they may become more aware of what it takes to make a strong proposal. For instance, functionality could be built into an extended chat system to support the indexical, elliptical and projective nature of group discourse.
Professional mathematics relies heavily upon inscription—the use of specialized notation, the inclusion of explicit statements of all deductive steps and the format of the formal proof—to support the discussion of math proposals. This can take place on an informal whiteboard, a university blackboard or in an academic journal. Everything that is to be indexed in the discussion is labeled unambiguously. To avoid ellipsis, theorems are stated explicitly, with all conditions and dependencies named. The projection of what is to be proven is encapsulated in the form of a proof, which starts with the givens and concludes with what is proven. Perhaps most importantly, proposals for how to proceed are listed in the proof itself as theorems, lemmas, etc. All of the elements of this system are organized sequentially.
One could imagine a chat system supplemented with a window in which participants could maintain an informal list of their proposals in a format analogous to the steps of a proof. After Sup’s proposal, the list might look like figure 21-4. When Sup made a proposal in the chat, he would enter a statement of it in the proof window in logical sequence. He could cross out his own proposal when he felt it had been convincingly argued against by the group.

Figure 21-4 goes approximately here
Of course, there are many design questions and options for doing something like this. Above all, would students understand this functionality and would they use it? Here it is only meant to be suggestive. The idea is that important proposals that were made would be retained in a visible way and be shared by the group.
Another useful tool for group mathematics would be a shared drawing area. In the chat environment used by Sup, Pin and Avr, there was no shared drawing, but a student could create a drawing and send it to the others. Pin did this twelve minutes after the part of the interaction shown in the excerpt. Before the drawing was shared, much time was lost due to confusion about references to triangles and vertices. For math problems involving geometric figures, it is clearly important to be able to share drawings easily and quickly. Again, there are many design issues, such as how to keep track of who drew what, who is allowed to erase, how to point to items in the drawing and how to capture a record of the graphical interactions in coordination with the text chatting.
In our next iteration of the VMT project experiments, we will experiment with a chat system that allows students to link their postings explicitly to other postings, to items in the shared drawing system and to elements of a proposals list. The links will appear as lines connecting the currently selected posting to the items that its author linked it to. Because postings may be linked to the statements that they respond to, the chat can optionally be displayed as a threaded discussion, avoiding some of the “chat confusion” that comes from postings appearing in a chronological order that obscures their response structure.
In general, mathematical group cognition may benefit from software features that support indexicality through displayed linking, shared drawings and threaded displays; compensate for ellipsis by maintaining memory lists that can be referenced; and aid projecting by making the status of open proposals explicit. In addition, ideas that were explored in the software prototypes of part I of this book can be applied to chat environments for group cognition; carefully selecting people to work together in groups, merging interpretive perspectives and structuring knowledge negotiation.
Online methods tend to parallel face-to-face methods, but technical mediation makes a difference, making some things easier (like working in parallel) and other things harder (reconciling computational differences, repairing losses from overlap). Design of the socio-technical mediation based on analysis of group interaction can improve the likelihood of desirable collaborative knowledge building. Whether face-to-face or online, the flow of the discourse from one proposal to the next, from method to method and from problem to strategy to sub-problem to solution defines the logic of mathematical group discourse—a unique form of group cognition.
The Rationality of Group Discourse
The science of conversation analysis discovered the sequential order inherent in ordinary talk (Sacks et al., 1974). Ethnomethodology explored the sense of accountability produced by conversational moves (Garfinkel, 1967). The concepts of sequentiality and accountability are closely related to the definition of rationality and high-level thought. This becomes particularly clear in mathematical discourse.
Mathematics can be considered a model of rational cognition. In modern times, logic itself has become a sub-domain of mathematics. The form of the mathematical proof is held up as a paradigm of pure thought.
A proof combines its own special forms of sequentiality and accountability. A proof begins with known facts (the givens of a problem), proceeds step-by-step through a rigorously defined sequence of propositions that are either themselves axioms (true by definition), results of previous proofs (e.g., theorems) or logical consequences of preceding steps. Each step is accountable—the person who proposes it must be prepared to demonstrate that it is true by virtue of being an axiom, theorem or consequence. The interesting part of the proof is not the individual propositions that make it up, but their strategic sequential flow from the givens to that which is to be proven (QED).
In the triangles problem discussed above, the mathematically interesting feature is neither the given values of the sides of the smaller triangles nor the answer of the length of the larger triangle, but the way one goes from the former to the latter: whether by elegant proportional reasoning, efficient algebraic manipulation or brute-force numeric computation. All of these strategies, in order to be considered mathematically valid, must adhere to a mathematical version of accountable sequentiality.
The difference in sequentiality and accountability between normal conversation and mathematical logic is one of degree. Logic is an abstraction from the flow of conversation, and proof is derivative from how we hold our conversational partners accountable to certain expectations of sense making. Thought is speech turned inward; the standards of logical cognition are derived from the patterns of social intercourse—rationality is derived from rationalization, not vice versa.
In the SimRocket transcript of chapter 12, we saw that the meaning of fragmentary utterances resulted from the sequentiality of their responsiveness to each other and to their strategic situation. Brent, in proposing that one pair of rockets was “different,” was thereby made accountable for convincing the other group members that what he said made sense in the context. This, in turn, led to the group recognizing a different sequentiality (called “paired configurations” in chapter 13) to the rocket descriptions in the simulation list.
The mathematical discourse of the chat log in this chapter (figure 21-2) followed quite closely the sequential format of a proof. Student proposals were strategic steps in a proof that would, if successfully completed, derive an answer to the problem from its givens (see figure 21-4). The accountability of each step meant that the group could not continue after a proposal was made until that proposal was accepted (with the second part of a proposal adjacency pair). Permissible responses to a proposal were to accept it because one recognized it as legitimate, to reject it, or to question it. If one rejected it, then the rejecter was accountable for providing a convincing reason for rejection. The epistemic status of a proposal was determined by the group through a negotiation method (similar to that in chapter 9’s model of collaborative knowledge building). Candidate proposals were offered to the group from individual perspectives as tentative beliefs or, better, as questions posed to the group. The determination of a proposal as knowledge had to be made by the group—by a response in the name of the group to a proposal addressed to the group. Of course, such knowledge is always subject to future critique and revision, but is in the meantime taken as knowledge that can be built upon.
Part of learning math is learning to enact methods that meet the standards of sequentiality and accountability. As we saw, Avr and Pin were much more skilled than Sup at participating in and contributing to such group methods. The group process progressed through multiple sequences of math proposal adjacency pairs building upon each other systematically. This group process of math cognition formally parallels at the group level the classic Socratic dialog method of math instruction in the Meno (Plato, 350 BC/1961), frequently imitated by classroom teachers (Lemke, 1990).
Considered at the small-group unit of analysis, the groups of students discussed in this chapter accomplished cognitive achievements. The groups underwent cognitive change and they solved math problems. They reflected upon and selected problem-solving strategies. They built knowledge and fashioned symbolic artifacts. They thought as groups. Viewing their behavior as a thinking group constituted by interacting interpretive individuals, we could make visible how shared meaning was constructed. Rather than focusing on hidden processes of individual cognition, we analyzed the group discourse and looked at issues of sequentiality, accountability, sociality and shared meaning making through the negotiation and acceptance of proposals. Many of the questions concerning mathematics education and thinking that arise for individual students presented themselves at the small-group unit of analysis as well.
If we accept that sequentiality and accountability are the hallmarks of high-order rational thought, then we see that group discourse meets the criteria for being considered an important form of cognition. Moreover, we have reason to hope that computer-supported collaboration can produce high-order cognitive achievements that rival and ultimately surpass those of individuals. This is not to deny the problems that can arise in groups, which may even hold back individual accomplishments. Nor is it to claim that current technologies provide the required forms of support. But it does point to a potential that transcends the limitations of the individual human mind and allows people to think together, to collaborate online in ways that effectively mediate group cognition.
Group Cognition and Distributed Cognitions
The notion of group cognition developed here may be considered a strong form of distributed cognition. It goes considerably beyond Norman’s (1993) argument that the individual mind extends outside the head to artifacts in the world as forms of external memory, like a reminder string on the finger. Many discussions of distributed cognition (as surveyed by Perkins, 1993) start from the individual and gradually add on elements of the person’s physical environment as cognitive aids or factors. Artifacts that extend perception (like the tip of a blind person’s cane) are often considered first, followed by external memory devices (notes on scraps of paper) or computational tools (calculator). A danger of this approach is that one is always tempted to fall back on the individual mind as the central locus of cognition.
The concept of group cognition in this book takes the collaborative interaction as starting point, e.g., a math proposal adjacency pair. The collective discourse is primary, and the individual appears as a node of the group, a contributor of pieces of the whole and an interpreter of the shared meaning. An individual act that is not part of a group process—for instance an utterance that is ignored by the group—is not a core part of the cognition, it does not contribute to the problem solving, knowledge building or meaning making. The search for the nature of collaborative knowledge building motivated by the breakdowns in the software studies of part I, culminated in the discovery in part II that the knowledge created by the boys working with the SimRocket program was constructed at the group-level discourse. This was paralleled in the chat log analysis in this chapter, where the problem solving was seen to be accomplished through adjacency pairs in the group discourse. The sense making occurs at the group unit of analysis and cannot be reduced to the individual level without losing its meaning. “Proposal?” remained an individual act—and was therefore consigned to irrelevance.
The view of meaning making as a group achievement is adopted from the social sciences, particularly ethnomethodology and conversation analysis. In the context of this book, the focus on group discourse is reflected upon as a methodological principle and the implications are drawn out for a theory of computer-supported collaboration.
This approach is closely related to what is called “distributed cognition” within the academic literature of CSCW, CSCL and the learning sciences. But distributed cognition is still a contentious term. Pea (1993b) proposed a version that is compatible with group cognition. For instance, he recognized the relation of knowledge building to the merging of perspectives at the group level when he said,
Knowledge is commonly socially constructed, through collaborative efforts toward shared objectives or by dialogues and challenges brought about by difference in persons’ perspectives. (p. 48)
He preferred the term “distributed intelligence” to “distributed cognition” because he was interested in how intelligent behavior is accomplished with resources that are distributed across people, environments and situations. He did not want to attribute cognition to the tools involved—just designed intelligence. Because the analysis of group cognition in this book is primarily concerned with the distribution of knowledge-building activities across people, Pea’s concern is less relevant—as discussed in chapter 19 where it is argued that groups of people can think even though computers cannot.
In an edited volume of papers that helped to popularize the concept of distributed cognition (Salomon, 1993a), the editor felt moved to defend individual cognition against the theory that his book was promoting (Salomon, 1993b):
The individual has been dismissed from theoretical considerations, possibly as an antithesis to the excessive emphasis on the individual by traditional psychological and educational approaches. (p. 111)
It should be clear that the conception of group cognition developed in this book has paid careful explicit attention to the role of the individual (from personal perspectives in part I, to parallel collaboration in part II, to interpretation in part III), without, however, allowing group phenomena to be reduced to the individual unit of analysis. It is both necessary and difficult to protect against reductionism precisely because of excessive emphasis in the past. One could accept the fall-back position offered by Salomon that this book’s focus on the group is a one-sided corrective, albeit one that is particularly appropriate in that the book has collaboration as the object of its analysis. However, to do so would be to underestimate the power of the group cognition view, allowing it to be conceived as a secondary effect, when in fact it is individual cognition that is the subsidiary product.
Salomon himself unintentionally provides a series of examples of how easy it is to slide back into the traditional outlook. His paper is a very carefully structured text, with three major points, and four arguments to the first of his points. In the first of these arguments, claiming that people’s cognitions in daily life are not always distributed, he writes, “Sitting at my desk thinking about the arguments I want to present in this chapter, I cannot but rely on my own cognitive repertoire; there is little in this austere surrounding that affords much cognitive distribution of any notable quality” (p. 114). This sentence could have been written by Descartes himself 400 years ago. Salomon’s chapter was surely developed on a computer surrounded by printouts of chapter outlines, notes, books and other chapters. He explicitly cites 41 published works—were they all in his head or were they in his austere surrounding? But more importantly, his entire thinking is completely situated in the circle of debates, positions and controversies to which his edited volume contributed. The concepts of cognition that he is thinking about could not be thought without the words, claims and issues that other people contributed. Even if, for the moment, he is only actively using resources from this complex social process that he managed to internalize, his thoughts cannot be separated from their community context. Furthermore, the very reason he is engaging in these private cogitations is in order to present his chapter to the public that is engaged with these issues. His social identity, his job and his collaboration with the other contributors to his volume are all at work. His individual thinking is but one narrow analytic perspective within the group cognition that produced his whole book.
For his second argument, Salomon makes use of Searle’s Chinese room argument (see chapter 19). He turns Searle’s argument around to argue that joint systems cannot have distributed understanding, only the person inside the box can. While this may apply to systems of external memories and external representations, as Pea suggested, the argument does not apply to groups of people, each of whom can intend meanings. This argument raises more problems for traditional cognitive scientists, who are focused on representations, than it does for group cognition.
In Salomon’s third argument, he tries to rescue a role for individual mental representations after acquiescing to the basic position of situated cognition from Heidegger as summarized by Winograd and Flores (1986). He renders the concept of “thrownness”—a cornerstone of Heidegger’s analysis of human temporality—in an explicitly atemporal way, and then picks up on how we gradually build up an understanding after a breakdown. This is a flawed gloss on Winograd and Flores’ summary of Heidegger’s theory of situated interpretation (see chapter 4). Salomon (1993b) interprets it within his own set of very non-Heideggerian assumptions:
This understanding can be stored only as a representation that will be brought to the fore when a subsequent “concernful action” of ours with the system breaks down, requiring us to reflect again. One would expect such reflection, based as it is on representations, … (p. 119)
Winograd and Flores present Heidegger’s philosophy precisely to show that one can have a coherent theory of human understanding without relying on representations. Yet Salomon assumes here that understanding “can be stored only as a representation” and that reflection must be based on representation, rather than being a hermeneutic process of situated interpretation. With the help of this misconstrual, Salomon reaches the conclusion he was aiming for: “one cannot but consider the role that individuals’ representations play” (p. 119). He assumes the centrality of the role of the individual as well as the role of representations. In contrast, we saw in chapter 13 that a group of students could reflect upon a breakdown situation within a group discourse, requiring neither representations nor individual reflections.
Again, in the fourth argument, Salomon assumes what is to be proven. He refers to situations in family therapy and organizational change, where group interaction is clearly central, and he states that “change is brought about by affecting the cognitions or behaviors of one or another member” (p. 119). But the whole point of something like family therapy is that the problems are systemic and the system as such must change in order to overcome the problems that present themselves through the individuals. What the therapist has to transform is precisely the nature of the interactions at the group unit of analysis: that is why it is called family therapy, not family-member therapy.
The preceding look at Salomon’s chapter demonstrates how hard it is for people steeped in Western cognitive theories—particularly in the traditions of education and psychology—to accept the principles and approaches of distributed, situated, group cognition and how easy it is for them to fall back into mentalist assumptions about individual thought and learning.
Writers from traditions of pragmatism (Dewey & Bentley, 1949/1991; Mead, 1934/1962), German philosophy (Habermas, 1981/1984; Hegel, 1807/1967; Heidegger, 1927/1996; Marx, 1867/1976), ethnomethodology (Dourish, 2001; Garfinkel, 1967; Sacks, 1992; Schegloff, 1991b), anthropology (Donald, 1991; Geertz, 1973; Hutchins, 1996; Lave, 1991) and dialogism (Bakhtin, 1986a; Harre & Gillet, 1999; Levinas, 1974/1998; Linell, 2001; Wegerif, 2004; Wells, 1999) seem much more comfortable thinking at the group unit of analysis. But most of them are not much concerned with the design of computer support for collaborative learning. I have tried to apply some of their thinking to examples that are of relevance to CSCL; to draw out their consequences for a methodology for studying computer-supported collaboration; and to sketch a theory of collaborative knowledge building. I have entitled the result “group cognition”—in order to show its relatedness to distributed cognition while differentiating it from varieties of distributed cognition that are more focused on the use of external memory artifacts than on thinking at the group unit of analysis. While the focus of many discussions of distributed cognition is on tacit understanding and skillful use of artifacts by individuals, I have specifically explored scientific reasoning and mathematical argumentation by small groups.
Future Work on Understanding Group Cognition
This book has tried to pose some fundamental questions about how to understand the mechanisms and potentialities of group cognition and to suggest ways to take on the task of responding to these issues through empirical studies. The Virtual Math Teams project has been organized as one small effort in this direction. The goal of the project is to identify and analyze common practices of online collaborative mathematical problem solving at the level of beginning algebra or geometry. What interactional methods do group members engage in to do math? How are these methods proposed, negotiated and shared? This analysis is oriented toward informing the design of collaboration environments for supporting math practices—including the design of software, training, curriculum, scaffolding, facilitation, feedback, etc.
The VMT project proceeds through iterative cycles of design-based research. We started with a simple commercially available chat system that was already familiar to many students. We conducted a number of small-group sessions of math problem solving using that chat system and observed what took place, including the chat log excerpt discussed earlier in this chapter.
We are acting as both analysts of computer-mediated collaborative math methods and designers of online environments to support communities of math discourse. The design-based research approach requires these perspectives to work in a coordinated and unified way. We are using—in tandem—both qualitative methods like conversation analysis and quantitative methods involving a multi-dimensional coding scheme to conduct rigorous and detailed analyses of chats and to compare results across chats. As we gain insight into how to make improvements, we will modify the software support system, the design of the service, the kinds of scaffolding provided, the training of group participants and leaders, the character of the math problems and the procedures for forming small groups. We have already added a shared whiteboard to allow the groups to make drawings. We plan to improve the drawing and pointing capabilities and add functionality to the chat to make it easier for students to be successful in making proposals to the group—adding explicit graphical support for referencing parts of a drawing or previous postings from a new posting and including a persistent list of proposals offered. As we deepen our understanding of effective student methods of “doing” collaborative online math, we will evolve the VMT service. We will also collect examples of effective and ineffective group cognition, and refine the theory of technology-mediated collaboration.
The VMT project is pursuing the empirical investigation of issues raised explicitly and implicitly in this book. We are analyzing the methods small groups use in collaborating with technology and designing technology to support the effective use of those methods. We are exploring the methods that teams of students use to discuss mathematics and are building communities for such discourse. We are fleshing out a theory of how group discourse mediates the collaboration of individuals in communities and using this theory to guide our practice.
The commercial software that we have used to support chat provides generic communication support. It was designed primarily for informal chatting and socializing or for coordination of schedules, not particularly for the interaction activities of collaborative knowledge building. Our conversation analysis of online math problem solving is starting to uncover questions that may suggest specific forms of software support for group cognition. How does a small group
Š initiate their interaction; form into a group; establish intersubjectivity?
Š decide upon a joint task to undertake; define and refine the task?
Š start to undertake the task; make proposals?
Š negotiate; persuade; question; explain?
Š adopt roles; socialize; define identities?
Š change activities; take turns; terminate interaction?
This chapter has suggested ways of proceeding beyond what has been said in this book. Avr’s opening proposal in the chat log excerpt provides a nice example of the initiation of group cognition that could be replicated and further analyzed: “Okay, I think we should start with the formula for the area of a triangle.” This proposal in the group chat explicitly relates an individual perspective (“I think”) to a group action (“we should start”) and to a community artifact (“the formula”). The posting constitutes the group as an agent (“we should start”) as well as positing the group as recipient of the proposal and, therefore, anticipating that the group itself should respond through one of its members. The posting can also be taken as an element of an already existing group activity, the chat. As such, the proposal partakes in the on-going mediation between the individuals participating in the chat and the institutions or communities-of-practice of school mathematics that provide the content, goals and context of the chat. The proposal—by instantiating a method of doing math in a specific technological medium—illustrates how group discourse mediates between participants and their culture. Avr’s proposal, “I think, we should … ,” thereby contrasts starkly with Descartes’ “I think, therefore I am,” which failed to escape the solipsistic individual perspective to constitute a collectivity.
The discourse-based conception of group cognition as proposed here rejects Descartes’ notion of thinking as a mental activity of individuals. Methodologically, it suggests looking in great detail at fine-grained interactions, lasting perhaps a minute or less, within groups. Rather than looking for learning effects that may affect an individual for a lifetime, it looks at brief episodes of meaning making that contribute to knowledge building within the group discourse as an activity. These episodes are characterized as “cognitive” because they display the requisite characteristics of sequentiality, accountability and sense making—not because they are extensions of individual cognition. Group cognition is a phenomenon at the group unit of analysis, not a derivative of either individual thinking or community-level establishment of cultural resources. It is the source of knowledge constructed collaboratively—and is therefore an appropriate foundation for CSCL and CSCW.
Individual learning enters the picture secondarily. Because collaboration requires shared understanding, processes of group cognition ensure that all participants keep pace with the group, to the extent needed for the group discourse’s practical purposes. This causes individuals to develop and alter their interpretations of constructed meaning and perhaps internalize cognitive artifacts based on the products of group cognition, such as meaningful texts.
Brent’s dramatic gesture, “This one’s different,” in chapter 12, like Avr’s opening proposal in this chapter, mediated through his individual interpretive perspective the group discourse dilemma and the scientific community. His contribution to the small-group interaction transformed shared meanings and uncovered for the group cognition a realm of differentiations belonging to the socio-cultural structure of the scientific world.
The exploration of more examples like Avr’s and Brent’s are needed to help to describe in both concrete and theoretical ways how group cognition is accomplished as a linguistic achievement. More rigorous conversational analysis of multiple chat studies will lead to an improved understanding of the methods that students use to constitute and structure group interaction and to engage in math problem solving. The VMT project is designed to generate and capture rich episodes of online group cognition as data for such exploration. The detailed interactional analysis of these episodes and their comparison to each other will increase our understanding of the following issues:
Š How is mathematics done by online small groups of students such that we can say, for instance, that the group is displaying deep mathematical understanding versus simply manipulating things algorithmically without such understanding?
Š What methods are used systematically by small groups in online, text-based environments for taking turns, keeping interaction flowing, repairing mistakes or misunderstandings, opening and closing sessions, constituting the group as a collectivity, etc.?
Š Can online events, activities and environments be designed to stimulate group cognition and to lower the barriers to participation and group success?
Š How can math discourse communities be catalyzed, grown and sustained by networks of small groups interacting with each other?
Addressing these issues will help to refine the notion of group cognition introduced in this book.
The Beginning
The quotation introducing chapter 9 made the point that as we build knowledge about something, the questions do not so much get answered, as get more and more complex. Perhaps this book has turned group cognition from a simple conundrum into a far more complex mystery. Hopefully, however, it has shown that it is a meaningful and important subject of inquiry, that we have just begun to probe.
The book started out from the promise of the Internet to overcome the limitations of individual cognition. Networked computers not only allow global access to information, but could also facilitate collaborative knowledge building within online communities. Case studies in the beginning of the book showed that even in virtual environments intentionally designed to support knowledge building, discussions were generally limited at best to the sharing of personal opinions. Commercial systems provide media for generic communication or transmission of information, but no specific support for the phases of more involved collaboration—such as those modeled in chapter 9. Driven by the market place demands of corporate users and educational institutions, the designs of these systems aim to structure and control individual access and usage rather than to scaffold group cognition.
Part I illustrated that computer support for collaborative knowledge building is a tricky undertaking. Software designs were explored there to provide support for group formation, interpretive perspectives and knowledge negotiation. The barriers to success that these investigations encountered were typical of CSCW and CSCL systems that try to establish innovative supports for group cognition.
The middle part of the book identified the need to better conceptualize collaborative knowledge building as a set of group processes. This led to the discovery of group cognition as a phenomenon of small-group discourse. Contributions to collaborative knowledge-building discussions do not typically express meanings that already existed in mental representations of individual participants. The utterances are indexical, elliptical and projective in the sense that they contribute to meaning at the group unit of analysis by virtue of their embeddedness in the group situation, discourse and activity. The meaning and the knowledge are originally constructed through group cognition. Individual cognition may later result from internalization or retrospective accounts. Accordingly, evidence of collaborative learning is to be found in the brief episodes of shared meaning making in which group knowing is constituted, rather than in traces of lasting capabilities of individuals, which are subject to numerous psychological factors.
Part II suggested the need for empirical research at a micro level of conversation analysis in order to determine how people actually form themselves into groups, intertwine personal, group and community perspectives and negotiate shared knowledge products. Example analyses in chapters 12 and 13 as well as in the present chapter illustrate the level of interpretation required to make visible the details of collaborative meaning making.
Part III drew upon these experiences to develop a notion of group cognition. It involves thinking at the small-group unit of analysis, where meaning is constructed that cannot be attributed to any individual, although it is constituted out of individual contributions and is reliant upon individual interpretations. Chapters in this part tried to overcome widespread resistance to the term, “group cognition,” and to suggest ways of conceptualizing collaborative processes that make it easier for us all to talk about group cognition.
In particular, conversation analysis was proposed as a methodology for making group cognition visible. Interpretations like those in chapters 12, 13 and 21 focus on thinking at the small-group unit of analysis. They look at intersubjective interactions like turn-taking, knowledge negotiation, adjacency pairs and conversational repair. Through such analysis, we see that the basic components of collaborative knowledge building are not actions of individuals, but are methods of small-group activity. Through them, shared meanings are proposed, adopted and refined. The processes of group cognition incorporate contributions by individuals, based on individual interpretations of the emerging and evolving group meanings. But these individual utterances are essentially fragmentary; they only become meaningful by virtue of their contributing to the group context. That is why computer support for collaborative knowledge building must be centrally concerned with group cognition.
The cycle of software prototyping, conversation analysis and theoretical reflection must be iterated repeatedly. Many innovations of CSCL and CSCW systems will have to be prototyped and tried out, developing a whole field of technology for use in supporting collaboration. The interactions that take place online in these and other contexts must be analyzed systematically, in order to catalog methods that people use to accomplish their group work, learning, communicating and thinking. The technology and the analyses should be conceptualized within a vocabulary adequate for making sense of them.
The comprehension of how thinking takes place at the small-group unit of analysis will guide the design of more effective computer support for collaborative knowledge building. Then the potential of group cognition can blossom around the world. This will require a global effort, itself a major instance of group cognition. The task of CSCL and CSCW has just begun.